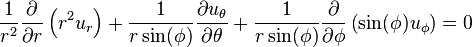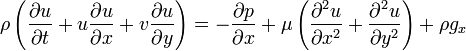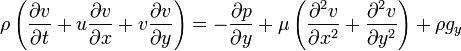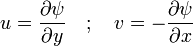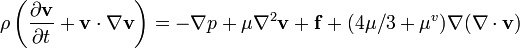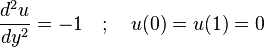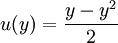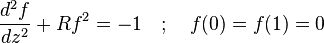Navier–Stokes equations
About this schools Wikipedia selection
This wikipedia selection has been chosen by volunteers helping SOS Children from Wikipedia for this Wikipedia Selection for schools. Do you want to know about sponsoring? See www.sponsorachild.org.uk
| Continuum mechanics |
|---|
 |
|
Laws
|
|
Solid mechanics
|
|
|
Rheology
|
The Navier-Stokes equations, named after Claude-Louis Navier and George Gabriel Stokes, describe the motion of fluid substances such as liquids and gases. These equations establish that changes in momentum in infinitesimal volumes of fluid are simply the sum of dissipative viscous forces (similar to friction), changes in pressure, gravity, and other forces acting inside the fluid: an application of Newton's second law to fluid.
They are one of the most useful sets of equations because they describe the physics of a large number of phenomena of academic and economic interest. They may be used to model weather, ocean currents, water flow in a pipe, flow around an airfoil (wing), and motion of stars inside a galaxy. As such, these equations in both full and simplified forms, are used in the design of aircraft and cars, the study of blood flow, the design of power stations, the analysis of the effects of pollution, etc. Coupled with Maxwell's equations they can be used to model and study magnetohydrodynamics.
The Navier-Stokes equations are also of great interest in a purely mathematical sense. Somewhat surprisingly, given their wide range of practical uses, mathematicians have not yet proven that in three dimensions solutions always exist ( existence), or that if they do exist they do not contain any infinities, singularities or discontinuities (smoothness). These are called the Navier-Stokes existence and smoothness problems. The Clay Mathematics Institute has called this one of the seven most important open problems in mathematics, and offered a $1,000,000 prize for a solution or a counter-example.
The Navier-Stokes equations are differential equations which, unlike algebraic equations, do not explicitly establish a relation among the variables of interest (e.g. velocity and pressure). Rather, they establish relations among the rates of change. For example, the Navier-Stokes equations for simple case of an ideal fluid (inviscid) can state that acceleration (the rate of change of velocity) is proportional to the gradient (a type of multivariate derivative) of pressure.
Contrary to what is normally seen in solid mechanics, the Navier-Stokes equations dictate not position but rather velocity. A solution of the Navier-Stokes equations is called a velocity field or flow field, which is a description of the velocity of the fluid at a given point in space and time. Once the velocity field is solved for, other quantities of interest (such as flow rate, drag force, or the path a "particle" of fluid will take) may be found.
Properties
Nonlinearity
The Navier-Stokes equations are nonlinear partial differential equations in almost every real situation (exceptions include one dimensional flow and creeping flow). The nonlinearity makes most problems difficult or impossible to solve and is part of the cause of turbulence.
The nonlinearity is due to convective acceleration, which is an acceleration associated with the change in velocity over position. Hence, any convective flow, whether turbulent or not, will involve nonlinearity, an example of convective but laminar (nonturbulent) flow would be the passage of a viscous fluid (for example, oil) through a small converging nozzle. Such flows, whether exactly solvable or not, can often be thoroughly studied and understood.
Turbulence
Turbulence is the time dependent chaotic behaviour seen in many fluid flows. It is generally believed that it is due to the inertia of the fluid as a whole: the culmination of time dependent and convective acceleration; hence flows where inertial effects are small tend to be laminar (the Reynolds number quantifies how much the flow is affected by inertia). It is believed, though not known with certainty, that the Navier-Stokes equations model turbulence properly.
Even though turbulence is an everyday experience, it is extremely difficult to find solutions, quantify, or in general characterize. A $1,000,000 prize was offered in May 2000 by the Clay Mathematics Institute to whoever makes preliminary progress toward a mathematical theory which will help in the understanding of this phenomenon.
The numerical solution of the Navier-Stokes equations for turbulent flow is extremely difficult, and due to the significantly different mixing-length scales that are involved in turbulent flow, the stable solution of this requires such a fine mesh resolution that the computational time becomes significantly infeasible for calculation (see Direct numerical simulation). Attempts to solve turbulent flow using a laminar solver typically result in a time-unsteady solution, which fails to converge appropriately. To counter this, several approximations such as the Reynolds averaged Navier-Stokes equations (RANS), supplemented with turbulence models (such as the k-ε model), are used in practical computational fluid dynamics (CFD) applications when modeling turbulent flows. Another technique for solving numerically the Navier-Stokes equation is the Large-eddy simulation (LES). This approach is computationally more expensive than the RANS method (in time and computer memory), but produces better results, since part of the turbulent characteristic scales are explicitly resolved.
Applicability
Together with supplemental equations (for example, conservation of mass) and well formulated boundary conditions, the Navier-Stokes equations seem to model fluid motion accurately; even turbulent flows seem (on average) to agree with real world observations.
The Navier-Stokes equations assume that the fluid being studied is a continuum. At very small scales or under extreme conditions, real fluids made out of discrete molecules will produce results different from the continuous fluids modeled by the Navier-Stokes equations. Depending on the Knudsen number of the problem, statistical mechanics or possibly even molecular dynamics may be a more appropriate approach.
Another limitation is very simply the complicated nature of the equations. Time tested formulations exist for common fluid families, but the application of the Navier-Stokes equations to less common families tends to result in very complicated formulations which are an area of current research. For this reason, the Navier-Stokes equations are usually written for Newtonian fluids.
Derivation and description
The derivation of the Navier-Stokes equations begins with the conservation of mass, momentum, and energy being written for an arbitrary control volume. In an inertial frame of reference, the most general form of the Navier-Stokes equations ends up being:
where  is the flow velocity,
is the flow velocity,  is the fluid density, p is the pressure,
is the fluid density, p is the pressure,  is the ( deviatoric) stress tensor, and
is the ( deviatoric) stress tensor, and 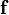 represents body forces (per unit volume) acting on the fluid and
represents body forces (per unit volume) acting on the fluid and  is the del operator. This is a statement of the conservation of momentum in a fluid and it is an application of Newton's second law to a continuum. This equation is often written using the substantive derivative, making it more apparent that this is a statement of Newton's law:
is the del operator. This is a statement of the conservation of momentum in a fluid and it is an application of Newton's second law to a continuum. This equation is often written using the substantive derivative, making it more apparent that this is a statement of Newton's law:
The left side of the equation describes acceleration, and may be composed of time dependent or convective effects (also the effects of non-inertial coordinates if present). The right side of the equation is in effect a summation of body forces (such as gravity) and spatial derivatives of surface forces (pressure and stress).
Convective acceleration
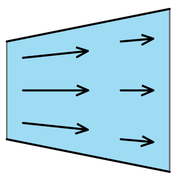
A very significant feature of the Navier-Stokes equations is the presence of convective acceleration. These terms describe the time independent acceleration of a fluid with respect to space, and are represented by the quantity:
The gradient of the velocity vector would more properly be written with the tensor derivative as 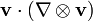 , where
, where 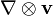 is the Jacobian matrix of velocity with respect to space. There are a few other ways to represent convection:
is the Jacobian matrix of velocity with respect to space. There are a few other ways to represent convection:
The second form above uses the advection operator and is common (note the difference between advection and convection — advection refers specifically to the transport of a scalar). The third form has use in irrotational flow, where the curl of the velocity (called vorticity) 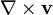 is absent.
is absent.
Regardless of what kind of fluid is being dealt with, convective acceleration is a nonlinear effect. Convection is present in most flows, exceptions include creeping flow and incompressible flow in one dimension.
Stresses
Stress in the fluid is represented by the 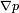 and
and 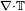 terms, these are gradients of surface forces, analogous to stresses in a solid.
terms, these are gradients of surface forces, analogous to stresses in a solid. 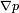 is called the pressure gradient and arises from normal stresses that turn up in almost all situations, dynamic or not.
is called the pressure gradient and arises from normal stresses that turn up in almost all situations, dynamic or not. 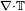 conventionally describes viscous forces; for incompressible flow, this is only a shear effect.
conventionally describes viscous forces; for incompressible flow, this is only a shear effect.
Interestingly, only the gradient of pressure shows up, not the pressure itself. The effect of the pressure gradient is that fluid flows from high pressure to low pressure.
The stress term 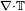 contains too many unknowns to be immediately usable, hence the general form above is not directly applicable to practical problems. For this reason, assumptions on the specific viscous behaviour of a fluid are made (based on natural observations) and applied in order to specify this quantity in terms of familiar variables, such as velocity. For example, this term becomes the useful quantity
contains too many unknowns to be immediately usable, hence the general form above is not directly applicable to practical problems. For this reason, assumptions on the specific viscous behaviour of a fluid are made (based on natural observations) and applied in order to specify this quantity in terms of familiar variables, such as velocity. For example, this term becomes the useful quantity 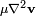 when the fluid is assumed incompressible and Newtonian.
when the fluid is assumed incompressible and Newtonian.
Other forces
 represents "other" ( body force) forces. Typically this is only gravity, but may include other fields (such as electromagnetic). In a non-inertial coordinate system, other "forces" such as that associated with rotating coordinates may be inserted.
represents "other" ( body force) forces. Typically this is only gravity, but may include other fields (such as electromagnetic). In a non-inertial coordinate system, other "forces" such as that associated with rotating coordinates may be inserted.
Often, these forces may be represented as the gradient of some scalar quantity. Gravity in the  direction, for example, is the gradient of
direction, for example, is the gradient of 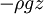 . Since pressure shows up only as a gradient, this implies that solving a problem without any such body force can be mended to include the body force by modifying pressure.
. Since pressure shows up only as a gradient, this implies that solving a problem without any such body force can be mended to include the body force by modifying pressure.
Other equations
The Navier-Stokes equations are strictly a statement of the conservation of momentum. In order to fully describe fluid flow, more information is needed (how much depends on the assumptions made), this may include boundary data ( no-slip, capillary surface, etc), the conservation of mass, the conservation of energy, and/or an equation of state.
Regardless of the flow assumptions, a statement of the conservation of mass is generally necessary. This is achieved through the mass continuity equation, given in its most general form as:
or, using the substantive derivative:
Incompressible flow of Newtonian fluids
The vast majority of work on the Navier-Stokes equations is done under an incompressible flow assumption for Newtonian fluids. The incompressible flow assumption typically holds well even when dealing with a "compressible" fluid, such as air at room temperature (even when flowing up to about Mach 0.3). Taking the incompressible flow assumption into account and assuming constant viscosity, the Navier-Stokes equations will read (in vector form):
f represents "other" body forces (forces per unit volume), such as gravity or centrifugal force. It's well worth observing the meaning of each term:
Note that only the convective terms are nonlinear for incompressible Newtonian flow. The convective acceleration is an acceleration caused by a (possibly steady) change in velocity over position, for example the speeding up of fluid entering a converging nozzle. Though individual fluid particles are being accelerated and thus are under unsteady motion, the flow field (a velocity distribution) will not necessarily be time dependent.
Another important observation is that the viscosity is represented by the vector Laplacian of the velocity field. This implies that Newtonian viscosity is diffusion of momentum, this works in much the same way as the diffusion of heat seen in the heat equation (which also involves the Laplacian).
If temperature effects are also neglected, the only "other" equation (apart from initial/boundary conditions) needed is the mass continuity equation. Under the incompressible assumption, density is a constant and it follows that the equation will simplify to:
This is more specifically a statement of the conservation of volume (see divergence).
These equations are commonly used in 3 coordinates systems: Cartesian, cylindrical, and spherical. The Cartesian equations follow directly from the vector equation above, obtaining equations in other coordinate systems will require a change of variables.
Cartesian coordinates
Writing the vector equation explicitly,
Note that gravity has been accounted for as a body force, and the values of 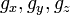 will depend on the orientation of gravity with respect to the chosen set of coordinates.
will depend on the orientation of gravity with respect to the chosen set of coordinates.
The continuity equation reads:
Note that the velocity components (the dependent variables to be solved for) are  ,
, 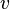 ,
, 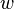 . This system of four equations comprises the most commonly used and studied form. Though comparatively more compact than other representations, this is a nonlinear system of partial differential equations for which solutions are difficult to obtain.
. This system of four equations comprises the most commonly used and studied form. Though comparatively more compact than other representations, this is a nonlinear system of partial differential equations for which solutions are difficult to obtain.
Cylindrical coordinates
A change of variables on the Cartesian equations will yield the following momentum equations for r, θ, and z:
The gravity components will generally not be constants, however for most applications either the coordinates are chosen so that the gravity components are constant or else it is assumed that gravity is counteracted by a pressure field (for example, flow in horizontal pipe is treated normally without gravity and without a vertical pressure gradient). The continuity equation is:
This cylindrical representation of the incompressible Navier-Stokes equations is the second most commonly seen (the first being Cartesian above). Cylindrical coordinates are chosen to take advantage of symmetry, so that a velocity component can disappear. A very common case is axisymmetric flow, where there is no tangential velocity (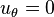 ) and the remaining quantities are independent of
) and the remaining quantities are independent of  :
:
Spherical coordinates
In spherical coordinates, the  ,
,  , and
, and  momentum equations are (note the convention used:
momentum equations are (note the convention used:  is colatitude):
is colatitude):
Mass continuity will read:
These equations could be (slightly) simplified by, for example, factoring 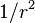 from the viscous terms. This isn't done to preserve the structure of the Laplacian and other quantities.
from the viscous terms. This isn't done to preserve the structure of the Laplacian and other quantities.
Stream function formulation
Taking the curl of the Navier-Stokes equation results in the elimination of pressure. This is especially easy to see if 2D Cartesian flow is assumed (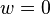 and no dependence of anything on
and no dependence of anything on  ), where the equations reduce to:
), where the equations reduce to:
Differentiating the first with respect to  , the second with respect to
, the second with respect to  and subtracting the resulting equations will eliminate pressure and any potential force. Defining the stream function
and subtracting the resulting equations will eliminate pressure and any potential force. Defining the stream function 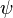 through
through
results in mass continuity being unconditionally satisfied (given the stream function is continuous), and then incompressible Newtonian 2D momentum and mass conservation degrade into one equation:
where  is the (2D) biharmonic operator and
is the (2D) biharmonic operator and 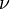 is the the kinematic viscosity. This single equation together with appropriate boundary conditions describes 2D fluid flow, taking only kinematic viscosity as a parameter. Note that the equation for creeping flow results when the left side is assumed zero.
is the the kinematic viscosity. This single equation together with appropriate boundary conditions describes 2D fluid flow, taking only kinematic viscosity as a parameter. Note that the equation for creeping flow results when the left side is assumed zero.
Compressible flow of Newtonian fluids
There are some exceptional phenomena that are closely linked with fluid compressibility. One of the obvious examples is sound. Description of such phenomena requires more general presentation of the Navier-Stokes equation that takes into account fluid compressibility. If viscosity is assumed a constant, one additional term appears, as shown here:
where μv is second viscosity coefficient. It is related to volume viscosity or bulk viscosity. This additional term disappears for incompressible fluid, when the divergence of the flow equals 0.
Application to specific problems
The Navier-Stokes equations, even when written explicitly for specific fluids, are rather generic in nature and their proper application to specific problems can be very diverse. This is partly because there is an enormous variety of problems that may be modeled, ranging from as simple as the distribution of static pressure to as complicated as multiphase flow driven by surface tension.
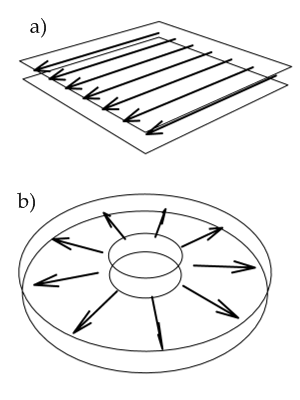
Generally, application to specific problems begins with some flow assumptions and initial/boundary condition formulation, this may be followed by scale analysis to further simplify the problem. For example, after assuming steady, parallel, one dimensional, nonconvective pressure driven flow between parallel plates, the resulting scaled (dimensionless) boundary value problem is:
The boundary condition is the no slip condition. This problem is easily solved for the flow field:
From this point onward more quantities of interest can be easily obtained, such as viscous drag force or net flow rate.
Difficulties may arise when the problem becomes slightly more complicated. A seemingly modest twist on the parallel flow above would be the radial flow between parallel plates; this involves convection and thus nonlinearity. The velocity field may be represented by a function  that must satisfy:
that must satisfy:
This ordinary differential equation is what is obtained when the Navier-Stokes equations are written and the flow assumptions applied (additionally, the pressure gradient is solved for). The nonlinear term makes this a very difficult problem to solve analytically (a lengthy implicit solution may be found which involves elliptic integrals and roots of cubic polynomials). Issues with the actual existence of solutions arise for R > 22.609 (approximately), the parameter R being the Reynolds number with appropriately chosen scales. This is an example of flow assumptions losing their applicability, and an example of the difficulty in "high" Reynolds number flows.

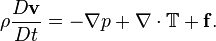
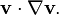

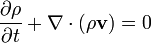
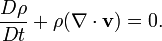

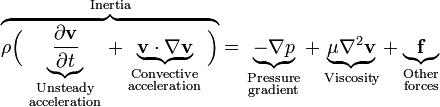
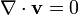
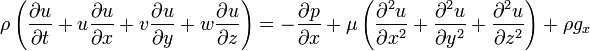

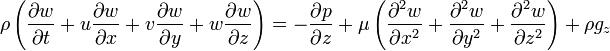
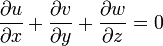
![\rho \left(\frac{\partial u_r}{\partial t} + u_r \frac{\partial u_r}{\partial r} + \frac{u_{\theta}}{r} \frac{\partial u_r}{\partial \theta} + u_z \frac{\partial u_r}{\partial z} - \frac{u_{\theta}^2}{r}\right) =
-\frac{\partial p}{\partial r} +
\mu \left[\frac{1}{r}\frac{\partial}{\partial r}\left(r \frac{\partial u_r}{\partial r}\right) + \frac{1}{r^2}\frac{\partial^2 u_r}{\partial \theta^2} + \frac{\partial^2 u_r}{\partial z^2} - \frac{u_r}{r^2} - \frac{2}{r^2}\frac{\partial u_{\theta}}{\partial \theta}\right] + \rho g_r](../../images/181/18167.png)
![\rho \left(\frac{\partial u_{\theta}}{\partial t} + u_r \frac{\partial u_{\theta}}{\partial r} + \frac{u_{\theta}}{r} \frac{\partial u_{\theta}}{\partial \theta} + u_z \frac{\partial u_{\theta}}{\partial z} + \frac{u_r u_{\theta}}{r}\right) =
-\frac{1}{r}\frac{\partial p}{\partial \theta} +
\mu \left[\frac{1}{r}\frac{\partial}{\partial r}\left(r \frac{\partial u_{\theta}}{\partial r}\right) + \frac{1}{r^2}\frac{\partial^2 u_{\theta}}{\partial \theta^2} + \frac{\partial^2 u_{\theta}}{\partial z^2} + \frac{2}{r^2}\frac{\partial u_r}{\partial \theta} - \frac{u_{\theta}}{r^2}\right] + \rho g_{\theta}](../../images/181/18168.png)
![\rho \left(\frac{\partial u_z}{\partial t} + u_r \frac{\partial u_z}{\partial r} + \frac{u_{\theta}}{r} \frac{\partial u_z}{\partial \theta} + u_z \frac{\partial u_z}{\partial z}\right) =
-\frac{\partial p}{\partial z} + \mu \left[\frac{1}{r}\frac{\partial}{\partial r}\left(r \frac{\partial u_z}{\partial r}\right) + \frac{1}{r^2}\frac{\partial^2 u_z}{\partial \theta^2} + \frac{\partial^2 u_z}{\partial z^2}\right] + \rho g_z](../../images/181/18169.png)
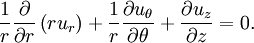
![\rho \left(\frac{\partial u_r}{\partial t} + u_r \frac{\partial u_r}{\partial r} + u_z \frac{\partial u_r}{\partial z}\right) =
-\frac{\partial p}{\partial r} +
\mu \left[\frac{1}{r}\frac{\partial}{\partial r}\left(r \frac{\partial u_r}{\partial r}\right) + \frac{\partial^2 u_r}{\partial z^2} - \frac{u_r}{r^2}\right] + \rho g_r](../../images/181/18172.png)
![\rho \left(\frac{\partial u_z}{\partial t} + u_r \frac{\partial u_z}{\partial r} + u_z \frac{\partial u_z}{\partial z}\right) =
-\frac{\partial p}{\partial z} + \mu \left[\frac{1}{r}\frac{\partial}{\partial r}\left(r \frac{\partial u_z}{\partial r}\right) + \frac{\partial^2 u_z}{\partial z^2}\right] + \rho g_z](../../images/181/18173.png)
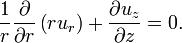
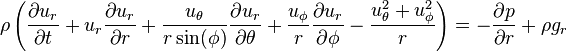
![\mu \left[
\frac{1}{r^2} \frac{\partial}{\partial r}\left(r^2 \frac{\partial u_r}{\partial r}\right) +
\frac{1}{r^2 \sin(\phi)^2} \frac{\partial^2 u_r}{\partial \theta^2} +
\frac{1}{r^2 \sin(\phi)} \frac{\partial}{\partial \phi}\left(\sin(\phi) \frac{\partial u_r}{\partial \phi}\right) -
2 \frac{u_r + \frac{\partial u_{\phi}}{\partial \phi} + u_{\phi} \cot(\phi)}{r^2} +
\frac{2}{r^2 \sin(\phi)} \frac{\partial u_{\theta}}{\partial \theta}
\right]](../../images/181/18176.png)

![\mu \left[
\frac{1}{r^2} \frac{\partial}{\partial r}\left(r^2 \frac{\partial u_{\theta}}{\partial r}\right) +
\frac{1}{r^2 \sin(\phi)^2} \frac{\partial^2 u_{\theta}}{\partial \theta^2} +
\frac{1}{r^2 \sin(\phi)} \frac{\partial}{\partial \phi}\left(\sin(\phi) \frac{\partial u_{\theta}}{\partial \phi}\right) +
\frac{2 \frac{\partial u_r}{\partial \theta} + 2 \cos(\phi) \frac{\partial u_{\theta}}{\partial \theta} - u_{\theta}}{r^2 \sin(\phi)^2}
\right]](../../images/181/18178.png)
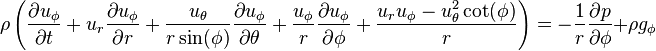
![\mu \left[
\frac{1}{r^2} \frac{\partial}{\partial r}\left(r^2 \frac{\partial u_{\phi}}{\partial r}\right) +
\frac{1}{r^2 \sin(\phi)^2} \frac{\partial^2 u_{\phi}}{\partial \theta^2} +
\frac{1}{r^2 \sin(\phi)} \frac{\partial}{\partial \phi}\left(\sin(\phi) \frac{\partial u_{\phi}}{\partial \phi}\right) +
\frac{2}{r^2} \frac{\partial u_r}{\partial \phi} -
\frac{u_{\phi} + 2 \cos(\phi) \frac{\partial u_{\theta}}{\partial \theta}}{r^2 \sin(\phi)^2}
\right]](../../images/181/18180.png)