Khan Academy on a Stick
Multiplying and factoring expressions
This topic will add a ton of tools to your algebraic toolbox. You'll be able to multiply any expression and learn to factor a bunch a well. This will allow you to solve a broad array of problems in algebra.
-
 Multiplying monomials
Multiplying monomials
Multiplying Monomials
-
 Dividing monomials
Dividing monomials
Dividing Monomials
-
 Multiplying and dividing monomials 1
Multiplying and dividing monomials 1
Multiplying and Dividing Monomials 1
-
 Multiplying and dividing monomials 2
Multiplying and dividing monomials 2
Multiplying and Dividing Monomials 2
-
 Multiplying and dividing monomials 3
Multiplying and dividing monomials 3
Multiplying and Dividing Monomials 3
-
 Monomial greatest common factor
Monomial greatest common factor
Monomial Greatest Common Factor
Multiplying and dividing monomials
"Monomials" sounds like a fancy word, but it just refers to single terms like "4x" or "8xy" or "17x^2z". In this tutorial, we'll learn to multiply and divide them using ideas you're already familiar with (like exponent properties and greatest common factor).
-
 Multiplying binomials word problem
Multiplying binomials word problem
Multiplying binomials word problem
-
 FOIL for multiplying binomials
FOIL for multiplying binomials
FOIL method for multiplying binomials
-
 Multiplying binomials with radicals
Multiplying binomials with radicals
Multiplying Binomials with Radicals
-
 Multiplying binomials and polynomials
Multiplying binomials and polynomials
Multiplying binomials
-
 FOIL method for multiplying binomials example 2
FOIL method for multiplying binomials example 2
FOIL method for multiplying binomials example 2
-
 Square a binomial
Square a binomial
Square a Binomial
-
 Special products of binomials
Special products of binomials
Special Products of Binomials
-
 Multiplying binomials to get difference of squares
Multiplying binomials to get difference of squares
Multiplying binomials to get difference of squares
-
 Squaring a binomial
Squaring a binomial
Squaring a binomial
-
 Squaring a binomial example 2
Squaring a binomial example 2
Squaring a binomial example 2
-
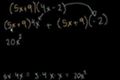 Classic multiplying binomials video
Classic multiplying binomials video
(Ax+By)(Ax+By)
Multiplying binomials
In this tutorial you'll learn that multiplying things like (4x-7)(-9x+5) just require the distributive property that you learned in elementary school. We'll touch on the FOIL method because it seems to be covered in a lot of schools, but we don't like it (we don't think it is good to memorize processes without knowing the why).
-
 Factoring and the distributive property 3
Factoring and the distributive property 3
Factoring and the Distributive Property 3
-
 Factoring linear binomials
Factoring linear binomials
-
 Factoring and the distributive property
Factoring and the distributive property
Factoring and the Distributive Property
-
 Factoring and the distributive property 2
Factoring and the distributive property 2
Factoring and the Distributive Property 2
-
 Factor expressions using the GCF
Factor expressions using the GCF
Factor expressions using the GCF
Factoring simple expressions
You already know a bit about multiplying expressions. We'll now reverse course and look at how to think about an expression as the product of simpler ones (just like we did when we find the factors of numbers).
-
 Factoring quadratic expressions
Factoring quadratic expressions
Factoring Quadratic Expressions
-
 Examples: Factoring simple quadratics
Examples: Factoring simple quadratics
A few examples of factoring quadratics
-
 Example 1: Factoring quadratic expressions
Example 1: Factoring quadratic expressions
Factoring trinomials with a leading 1 coefficient
-
 Example 1: Factoring trinomials with a common factor
Example 1: Factoring trinomials with a common factor
Factoring trinomials with a common factor
Factoring quadratic expressions
Not only is factoring quadratic expressions (essentially second-degree polynomials) fun, but it is good for you. It will allow you to analyze and solve a whole range of equations. It will allow you to impress people at parties and move up the career ladder. How exciting!
-
 Factoring special products
Factoring special products
Factoring Special Products
-
 Example 1: Factoring difference of squares
Example 1: Factoring difference of squares
Factoring difference of squares
-
 Example 2: Factoring difference of squares
Example 2: Factoring difference of squares
Factoring difference of squares
-
 Factoring to produce difference of squares
Factoring to produce difference of squares
-
 Example: Factoring perfect square trinomials
Example: Factoring perfect square trinomials
Factoring perfect square trinomials
-
 Example: Factoring a fourth degree expression
Example: Factoring a fourth degree expression
Factoring Special Products 2
-
 Example: Factoring special products
Example: Factoring special products
Factoring Special Products 1
Factoring special products
You will encounter very factorable quadratics that don't always seem so. This tutorial will expand your arsenal by exposing you to special products like difference-of-squares and perfect square quadratics.
-
 Factor by grouping and factoring completely
Factor by grouping and factoring completely
Factor by Grouping and Factoring Completely
-
 Example: Basic grouping
Example: Basic grouping
Factoring Trinomials by Grouping 1
-
 Example 1: Factoring by grouping
Example 1: Factoring by grouping
Factoring trinomials with a non-1 leading coefficient by grouping
-
 Example 2: Factoring by grouping
Example 2: Factoring by grouping
U09_L1_T2_we2 Factoring Trinomials by Grouping 2
-
 Example 3: Factoring by grouping
Example 3: Factoring by grouping
Factoring simple quadratic expressions
-
 Example 4: Factoring by grouping
Example 4: Factoring by grouping
Factoring Trinomials by Grouping 4
-
 Example 5: Factoring by grouping
Example 5: Factoring by grouping
Factoring Trinomials by Grouping 5
-
 Example 6: Factoring by grouping
Example 6: Factoring by grouping
Factoring Trinomials by Grouping 6
Factoring by grouping
Factoring by grouping is probably the one thing that most people never really learn well. Your fate doesn't have to be the same. In this tutorial, you'll see how factoring by grouping can be used to factor a quadratic expression where the coefficient on the x^2 term is something other than 1?
Factoring quadratics in two variables
We'll now extend the application of our quadratic-factoring toolkit, by factoring expressions with two variables. As we'll see, this is really just an extension of what you probably already know (or at least will know after working through this tutorial). Onward!
-
 Terms coefficients and exponents in a polynomial
Terms coefficients and exponents in a polynomial
Terms coefficients and exponents in a polynomial
-
 Interesting polynomial coefficient problem
Interesting polynomial coefficient problem
Finding the coefficients of a third degree polynomial given 2 roots and the y-intercept
-
 Polynomials 1
Polynomials 1
Polynomials 1
-
 Polynomials 2
Polynomials 2
Polynomials 2
-
 Evaluating a polynomial at a given value
Evaluating a polynomial at a given value
Evaluating a polynomial at a given value
-
 Simplify a polynomial
Simplify a polynomial
Working through simplifying a polynomial
-
 Adding polynomials
Adding polynomials
Adding Polynomials
-
 Example: Adding polynomials with multiple variables
Example: Adding polynomials with multiple variables
Basic example of simplifying a polynomial expression with multiple variables.
-
 Addition and subtraction of polynomials
Addition and subtraction of polynomials
Addition and Subtraction of Polynomials
-
 Adding and subtracting polynomials 1
Adding and subtracting polynomials 1
Adding and Subtracting Polynomials 1
-
 Adding and subtracting polynomials 2
Adding and subtracting polynomials 2
Adding and Subtracting Polynomials 2
-
 Adding and subtracting polynomials 3
Adding and subtracting polynomials 3
Adding and Subtracting Polynomials 3
-
 Subtracting polynomials
Subtracting polynomials
Subtracting Polynomials
-
 Subtracting polynomials with multiple variables
Subtracting polynomials with multiple variables
Subtracting polynomials with multiple variables
Polynomial basics
"Polynomials" sound like a fancy word, but you just have to break down the root words. "Poly" means "many". So we're just talking about "many nomials" and everyone knows what a "nomial" is. Okay, most of us don't. Well, a polynomials has "many" terms. From understanding what a "term" is to basic simplification, addition and subtraction of polynomials, this tutorial will get you very familiar with the world of many "nomials." :)
-
 Multiplying monomials by polynomials
Multiplying monomials by polynomials
Multiplying Monomials by Polynomials
-
 Multiplying polynomials example
Multiplying polynomials example
Multiplying Polynomials
-
 Multiplying polynomials example 2
Multiplying polynomials example 2
-
 Multiplying polynomials example 3
Multiplying polynomials example 3
More multiplying polynomials
Multiplying polynomials
You'll see in this tutorial that multiplying polynomials is just an extension of the same distributive property that you've already learned to multiply simpler expression (that's why we think FOIL is lame--it doesn't generalize and it is more memorization than real understanding).
 Factoring quadratics with two variables
Factoring quadratics with two variables Factoring quadratics with two variables example
Factoring quadratics with two variables example