Exponential and logarithmic functions : Khan Academy on a Stick

Khan Academy on a Stick

Algebra II

Exponential growth functions
Exponential Growth Functions
Graphing exponential functions
Graphing Exponential Functions
Understanding linear and exponential models
Comparing exponential and quadratic models
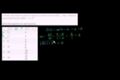Constructing linear and exponential functions from data
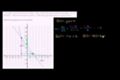Constructing linear and exponential functions from graph

Exponential growth and decay

From compound interest to population growth to half lives of radioactive materials, it all comes down to exponential growth and decay.

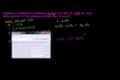Exponential growth and decay word problems
Word Problem Solving- Exponential Growth and Decay
Decay of cesium 137 example
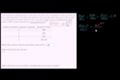Modeling ticket fines with exponential function
Solving exponential equation

Modeling with exponential functions

As we'll see in this tutorial, anything from compound interest to radioactive decay can be modeled with exponential functions.

Comparing exponential and logarithmic functions
Graphing logarithmic functions
Graphing Logarithmic Functions
Matching functions to their graphs
Graphs of logarithmic functions
Vi and Sal explore how we think about scale
Vi Hart (http://www.youtube.com/vihart) and Sal talk about how we humans perceive things nonlinearly

Logarithmic functions

This tutorial shows you what a logarithmic function is. It will then go on to show the many times in nature and science that these type of functions are useful to describe what is happening.

Solving logarithmic equations
Solving Logarithmic Equations
Solving logarithmic equations
Solving Logarithmic Equations

Logarithmic equations

Now that you are familiar with logarithms and logarithmic functions, let's think about how to solve equations involving logarithms.

Introduction to interest
What interest is. Simple versus compound interest.
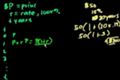Interest (part 2)
More on simple and compound interest
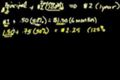Introduction to compound interest and e
Compounding interest multiple times a year.
Compound interest and e (part 2)
Compounding 100% annual interest continuously over a year converges to e (2.71...)
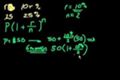Compound interest and e (part 3)
Continuously compounding $P in principal at an annual interest rate of r for a year ends up with a final payment of $Pe^r
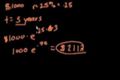Compound interest and e (part 4)
Continuously compounding for multiple years.

Continuous compounding and e

This tutorial introduces us to one of the derivations (from finance and continuously compounding interest) of the irrational number 'e' which is roughly 2.71...