Checked content

File:Pythag anim.gif

Pythag_anim.gif ‎(200 × 200 pixels, file size: 64 KB, MIME type: image/gif, looped, 31 frames, 6.8 s)
Mathematics icon This image was selected as picture of the month on the Mathematics Portal for October 2011
Did You Know This image appeared on Wikipedia's Main Page in the Did you know? column on 26 April 2013.
Wikipedia

Summary

Description
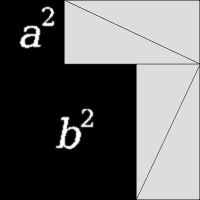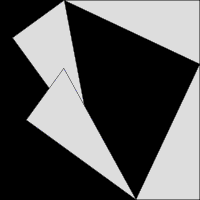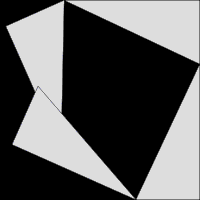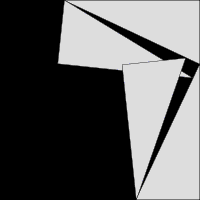
English: Animation of a proof of Pythagoras' theorem, showing how by rearranging triangles the areas a2 + b2 and c2 can be shown to be the same. The area of the outer square never changes, and the total area of the four right triangles is the same at both the beginning and the end, therefore the black area at the beginning, a2 + b2, must equal the black area at the end, c2. The angle of the triangles is arbitrary, therefore this works as a general proof. To be more explicit...


The situation at the start is:

Area_of_the_Outer_Square - (4 x Area_of_a_Triangle) = a2 + b2

...and the situation at the end is:

Area_of_the_Outer_Square - (4 x Area_of_a_Triangle) = c2

The values on both left-hand sides of these two equations are exactly the same (only positions have changed) therefore the values on the right-hand sides must also be exactly the same: a2 + b2 = c2.
Date
Source Own work
Author JohnBlackburne

Licensing

I, the copyright holder of this work, hereby publish it under the following licenses:
w:en:Creative Commons
attribution share alike		 This file is licensed under the Creative Commons Attribution-Share Alike 3.0 Unported license.
You are free:
  • to share – to copy, distribute and transmit the work
  • to remix – to adapt the work
Under the following conditions:
  • attribution – You must attribute the work in the manner specified by the author or licensor (but not in any way that suggests that they endorse you or your use of the work).
  • share alike – If you alter, transform, or build upon this work, you may distribute the resulting work only under the same or similar license to this one.
GNU head Permission is granted to copy, distribute and/or modify this document under the terms of the GNU Free Documentation License, Version 1.2 or any later version published by the Free Software Foundation; with no Invariant Sections, no Front-Cover Texts, and no Back-Cover Texts. A copy of the license is included in the section entitled GNU Free Documentation License.

You may select the license of your choice.

The following pages on Schools Wikipedia link to this image (list may be incomplete):

Metadata

This file contains additional information, probably added from the digital camera or scanner used to create or digitize it. If the file has been modified from its original state, some details may not fully reflect the modified file.
GIF file comment GifBuilder 1.1

Find out about Schools Wikipedia

Wikipedia for Schools was collected by SOS Children's Villages. SOS Childrens Villages is famous for the love and shelter it brings to lone children, but we also support families in the areas around our Children's Villages, helping those who need us the most. Have you thought about sponsoring a child?