 |
This is a file from the Wikimedia Commons. Information from its description page there is shown below.
Commons is a freely licensed media file repository. You can help.
|
Summary
| Description |
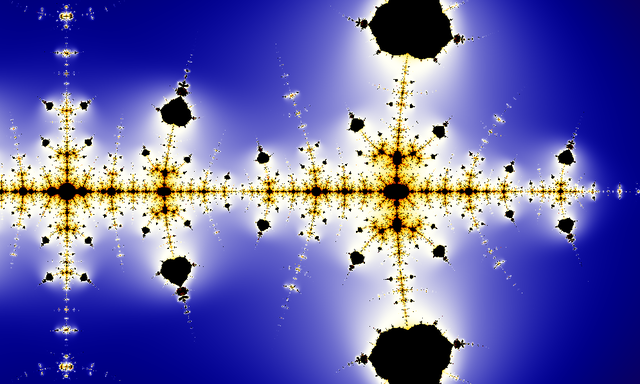
English: The Collatz map can be viewed as the restriction to the integers of the smooth real and complex map
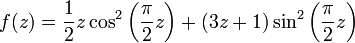 , ,
which simplifies to 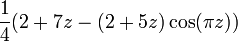 . If the standard Collatz map defined above is optimized by replacing the relation 3n + 1 with the common substitute "shortcut" relation (3n + 1)/2, it can be viewed as the restriction to the integers of the smooth real and complex map . If the standard Collatz map defined above is optimized by replacing the relation 3n + 1 with the common substitute "shortcut" relation (3n + 1)/2, it can be viewed as the restriction to the integers of the smooth real and complex map
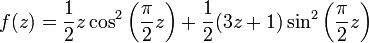 , ,
which simplifies to 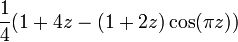 . . Iterating the above optimized map in the complex plane produces the Collatz fractal.
|
| Date |
21 October 2005 |
| Source |
English wikipedia |
| Author |
Pokipsy76 |
Licensing
| Public domainPublic domainfalsefalse |
 |
I, the copyright holder of this work, release this work into the public domain. This applies worldwide.
In some countries this may not be legally possible; if so:
I grant anyone the right to use this work for any purpose, without any conditions, unless such conditions are required by law.Public domainPublic domainfalsefalse
|
File usage
The following pages on Schools Wikipedia link to this image (list may be incomplete):
Schools Wikipedia was launched to make learning available to everyone. SOS Children is famous for the love and shelter it brings to lone children, but we also support families in the areas around our Children's Villages, helping those who need us the most. Have you heard about child sponsorship? Visit our web site to find out.

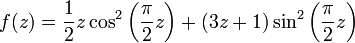 ,
,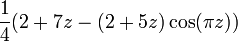 . If the standard Collatz map defined above is optimized by replacing the relation 3n + 1 with the common substitute "shortcut" relation (3n + 1)/2, it can be viewed as the restriction to the integers of the smooth real and complex map
. If the standard Collatz map defined above is optimized by replacing the relation 3n + 1 with the common substitute "shortcut" relation (3n + 1)/2, it can be viewed as the restriction to the integers of the smooth real and complex map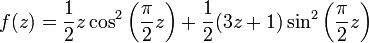 ,
,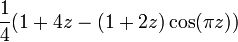 .
.
