Khan Academy on a Stick
Linear equations and inequalities
We will now equate two algebraic expressions and think about how it might constrain what value the variables can take on. The algebraic manipulation you learn here really is the heart of algebra.
-
Simple equations of the form ax = b
Let's ease into this, shall we? Here's an introduction to basic algebraic equations of the form ax=b. Remember that you can check to see if you have the right answer by substituting it for the variable!
-
Simple equations of the form x/a = b
Remember that what you do to one side, you have to do to the other. Will you multiply or divide both sides to dump the fraction, x/a? Let's do it together.
-
Simple equations of the form x + a = b
Here's another one-step equation to solve. Unlike the previous two that involved multiplication and division, this one adds a number to the variable. Which operation is opposite of addition?
-
Simple equations: examples solving a variety of forms
Some quick examples to practice solving a variety of one step equations. All 4 operations (add, subtract, multiple, divide) are paired with variables.
-
Solving a more complicated equation
This example demonstrates how we solve an equation expressed such: ax + b = c. It's a little more complicated than previous examples, but you can do it!
-
Example: two-step equations
Let's practice some two step equations, some of which require merging terms and using the distributive property.
-
Example: two-step equation with numerator x
Solve the equation by isolating the term with numerator x.
Solving basic equations
Now we'll introduce you to the most fundamental ideas of what equations mean and how to solve them. We'll then do a bunch of examples to make sure you're comfortable with things like 3x – 7 = 8. So relax, grab a cup of hot chocolate, and be on your way to becoming an algebra rockstar. And, by the way, in any of the "example" videos, try to solve the problem on your own before seeing how Sal does it. It makes the learning better!
-
Linear equation word problem
You'll find that solving linear equations has real world applications. Here's a word problem example that demonstrates that.
-
Word problem: solving equations
Here's a nifty word problem in which we find the dimensions of a garden given only the perimeter. Let's create an equation to solve!
-
Sum of consecutive odd integers
Linear equation word problems
Now that we are reasonably familiar with what a linear equation is and how we can solve them, let's apply these skills to tackling real-world problems.
-
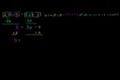Variables on both sides
Equations with the variable on both sides.
-
Example 1: Variables on both sides
Multi-step equations 1
-
Example 2: Variables on both sides
Solving Equations 2
-
Understanding steps when solving equations
-
Figuring out missing algebraic step
Solving fancier linear equations
You've been through "Equations for beginners" and are feeling good. Well, this tutorial continues that journey by addressing equations that are just a bit more fancy. By the end of this tutorial, you really will have some of the core algebraic tools in your toolkit!
-
Multiplying and dividing with inequalities
Our discussion of linear inequalities begins with multiplying and dividing by negative numbers. Listen closely for the word "swap." Super important!
-
Multiplying and dividing with inequalities example
In addition to solving the inequality, we'll graph the solution. Remember to swap if you mutiply both sides of the inequality by a negative number.
-
Constructing and solving a one-step inequality
Inequalities are more than abstract concepts and exercises. They help solve real life problems. Here's an example.
-
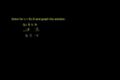One-step inequality involving addition
One-Step Inequalities
-
Inequalities using addition and subtraction
Inequalities Using Addition and Subtraction
-
Solving a two-step inequality
We're turning up the heat a little and asking you to help us solve a multi-step inequality problem.
-
Multi-step inequalities
Compound Inequalities
-
Multi-step inequalities 2
Multi-Step Inequalities 2
-
Multi-step inequalities 3
Multi-Step Inequalities
-
Constructing and solving a two-step inequality
We'll talk you through this fun and challenging inequality problem.
-
Constructing and solving a multi-step inequality example
Here's an opportunity for you to follow along with us as we interpret, construct, and solve a word problem inequality. It will be fun!
-
Constructing, solving two-step inequality example
Let's tackle this word problem together. We'll interpret the information and then construct a linear inequality to solve it.
Linear inequalities
In this tutorial you'll discover that much of the logic you've used to solve equations can also be applied to think about inequalities!
-
Writing proportions
As opposed to actually solving ratio word problems, in this video we'll practice just setting up the equations to solve them. Watch as we set up proportions to get at the answers.
-
Solve a proportion with unknown variable
Here's a great video where we explain the reasoning behind solving proportions. We'll put some algebra to work to get our answers, too.
-
Proportions 2 exercise examples
-
Constructing equations from proportions to solve problems
We're putting some nifty thinking to work as we use proportions to create equations which then tell us how much tip to leave for dinner.
-
Ratio problem with basic algebra
A slightly more involved ratio problem with algebra
Ratios and proportions
You remember a thing or two about ratios and proportions from your pre-algebra days. Well, how can we use these same ideas to solve problems in algebra? This tutorial re-introduces ratios in an algebraic context and helps us solve some awesome problems!
-
Direct and inverse variation
Understanding direct and inverse variation
-
Recognizing direct and inverse variation
Examples of variables varying directly and inversely
-
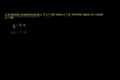Proportionality constant for direct variation
Proportionality Constant for Direct Variation
-
Direct variation 1
Direct Variation 1
-
Direct variation application
Direct Variation Application
Direct and inverse variation
Whether you are talking about how force relates to acceleration or how the cost of movie tickets relates to the number of people going, it is not uncommon in this universe for things to vary directly. Similarly, when you are, say, talking about how hunger might relate to seeing roadkill, things can vary inversely. This tutorial digs deeper into these ideas with a bunch of examples of direct and inverse variation.